could you please help with these questions
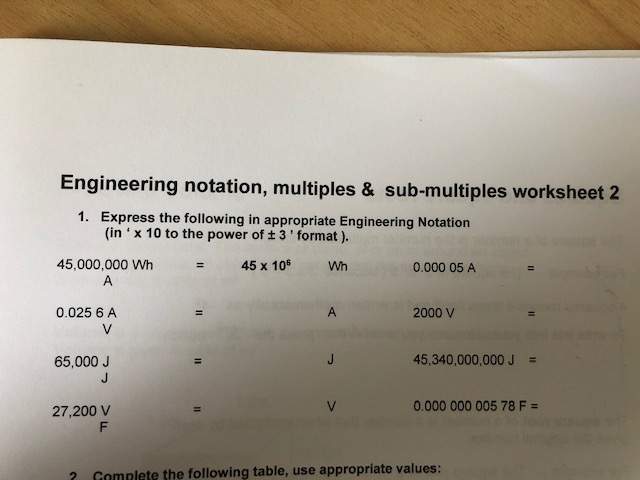
There has been come missed typesetting of this page. The "A" is related to the 0.00005 on the line above.
The objective of engineering notation is to convert any number \( c \) to one of the form \( m \times 10^{n} \), where \( m \) is a number from 1 to (just below) 1000, and \( n \) is an integer multiple of 3.
There are various ways of converting to Engineering notation. Your calculator may have a special key (an ENG button), but a method that works anywhere is this:
For numbers not in Scientific Notation and at least 1:
1. Start with the mantissa \( m \) equal to the original number, and the exponent \( n = 0 \). Group the digits into threes, starting from the right.
2. If \( m < 1000 \), skip to step 4. Otherwise, go to step 3.
3. Move the decimal point three places to the left in \( m \), and add 3 to \( n \). Go back to step 2.
4. You now have two numbers. One is the digits of the original number \( m \), and the other is \( n \).
For numbers not in Scientific Notation and less than 1:
1. Start with the mantissa \( m \) equal to the original number, and the exponent \( n = 0 \). Group the digits into threes, starting from the decimal point and going right.
2. If the last group has less than 3 digits, add trailing zeroes until it has 3 digits.
3. If \( m \geq 1 \), go to step 5. Otherwise, go to the next step.
4. Move the decimal point three places to the right in \( m \), and subtract 3 from \( n \). Go back to step 3.
5. You now have two numbers. One is the digits of the original number \( m \), and the other is \( n \).
You have your Engineering notation number as \( m \times 10^n \), where \( 1 \leq |m| < 1000 \) and \( n \) is a multiple of 3.
I will post some examples in the next post.
Thanks
Bart
Some examples:
Example 1:
45000000
1. 45000000 → 45,000,000. \( m \) = 45000000, \( n \) = 0.
2. \( m < 1000 \) → go to step 3
3. \( m = 45000 \), \( n = 3 \)
2. \( m \geq 1000 \) → go to step 3
3. \( m = 45 \), \( n = 6 \)
2. \( m < 1000 \) → go to step 4
4. \( m = 45 \), \( n = 6 \)
Therefore, \(45000000 = 45 \times 10^6 \)
Example 2:
0.0000000007
1. 0.0000000007 → 0.000 000 000 7. \( m \) = 0.0000000007, \( n \) = 0.
2. 0.000 000 000 7 → 0.000 000 000 700
3. \( m < 1 \) → go to step 4
4. \( m = 0.000000700 \), \( n = -3 \)
3. \( m < 1 \) → go to step 4
4. \( m = 0.000700 \), \( n = -6 \)
3. \( m < 1 \) → go to step 4
4. \( m = 0.700 \), \( n = -9 \)
3. \( m < 1 \) → go to step 4
4. \( m = 700 \), \( n = -12 \)
3. \( m \geq 1 \) → go to step 5
4. \( m = 700 \), \( n = -12 \)
Therefore, \(0.0000000007 = 700 \times 10^{-12} \)
Thanks
Bart
Thanks
Bart