Worksheet 1A - Basic Ohm's Law - Solutions and Commentary
Question 1
The objective of this question is to work out the unknown supply voltage \( V_{\mathrm{s}} \).
We have the following information:
- the current flow through the circuit (20 A); and
- the total resistance of the circuit (10 Ω).
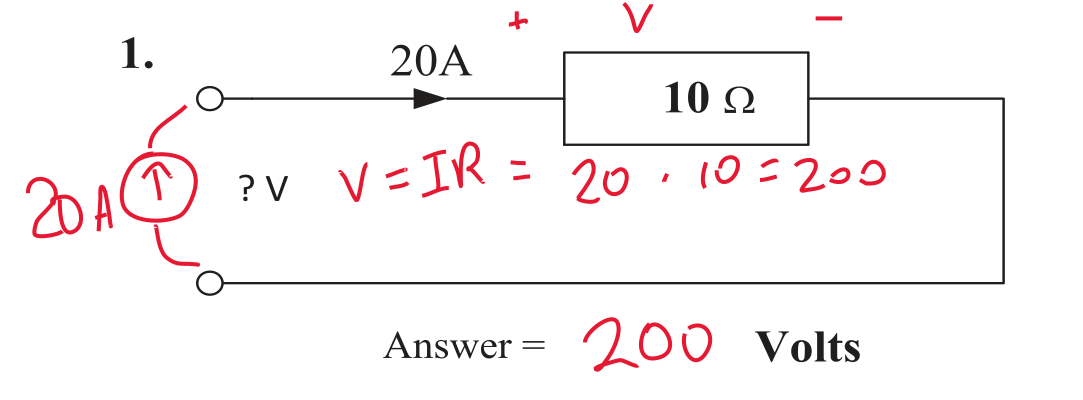
The driving source is depicted as a current source, as the current flow in the circuit is fixed. The current source is drawn as shown so that it produces a positive current in the same direction as the current shown. This circuit may be solved using Ohm's Law:
\( V_{\mathrm{s}} = IR = 20 \cdot 10 = 200 \) i.e. \( V_{\mathrm{s}}\) = 200 V.
Question 2
Question 2 is similar to Question 1, except we are asked to calculate the resistance \( R \).
We have the following information:
- the current flow through the circuit (19 A); and
- the voltage applied to the circuit (100 V).
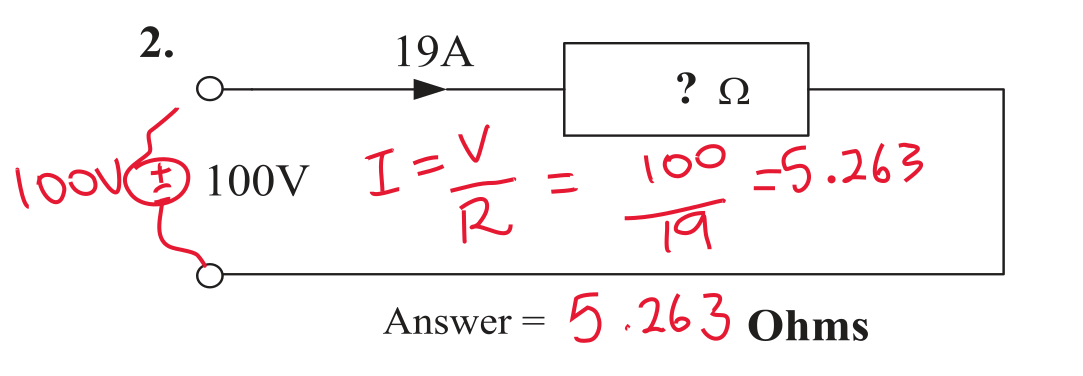
The driving source has been identified as a voltage source, as the voltage of the circuit is fixed. The positive terminal is as shown, because the polarity shown will produce a current flow in the direction shown. This circuit may be solved using Ohm's Law:
\( R = \frac{V}{I} = \frac{100}{19} \) i.e. \( R \) = 5.26 Ω (to 3 sf) #.
Question 3
Question 3 requires us to calculate the current \( I \).
We have the following information:
- the resistance of the circuit (36 Ω); and
- the voltage applied to the circuit (12 V).
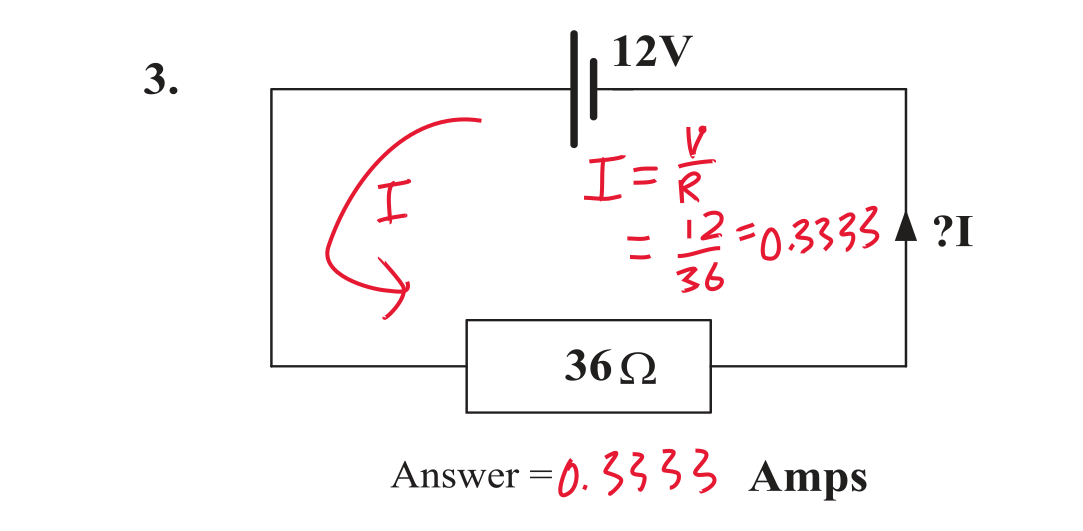
A voltage source is already shown, so no extra source is added. The flow of current is anti-clockwise, because the positive terminal is on the lower left. The polarity of the voltage drop across the resistor is as shown as this corresponds to a voltage "drop" across the resistor with the current flow counter-clockwise.
This circuit may be solved using Ohm's Law:
\( I = \frac{V}{R} = \frac{12}{36} \) i.e. \( I \) = 0.333 A (to 3 sf) #.
Question 4
Question 4 requires us to calculate the current \( I \), similar to Question 3.
We have the following information:
- the resistance of the circuit (240 Ω); and
- the voltage applied to the circuit (240 V).
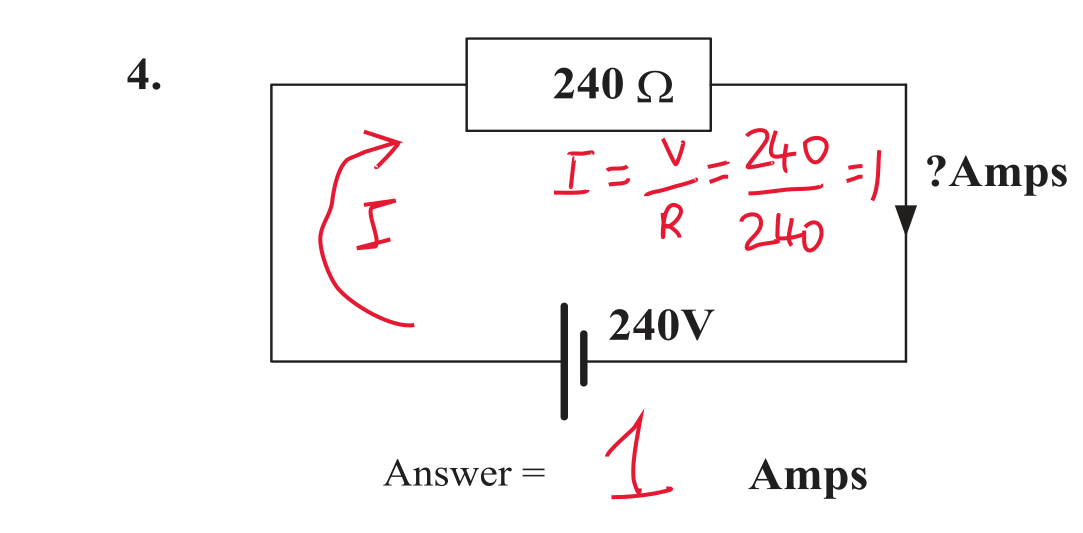
The direction of current flow is consistent with the polarity of the voltage source. This circuit may be solved using Ohm's Law:
\( I = \frac{V}{R} = \frac{240}{240} \) i.e. \( I \) = 1 A #.
Question 5
The objective of this question is to work out the unknown supply voltage \( V_{\mathrm{s}} \), similarly to Question 1.
We have the following information:
- the current flow through the circuit (1.5 A); and
- the total resistance of the circuit (5 Ω).
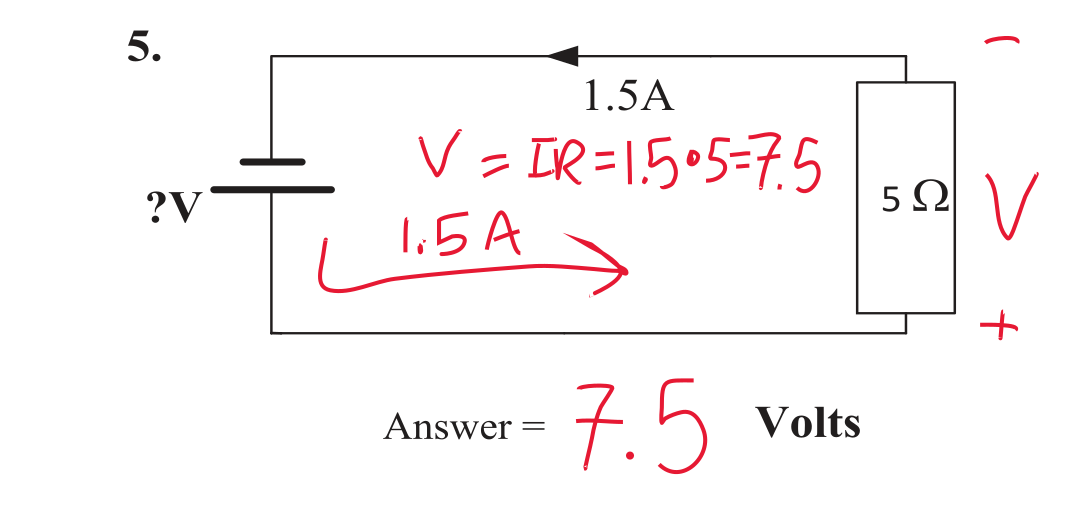
This circuit may be solved using Ohm's Law:
\( V_{\mathrm{s}} = IR = 1.5 \cdot 5 = 7.5 \) i.e. \( V_{\mathrm{s}}\) = 7.5 V #.
Question 6
This question requires us to fill out a table of unknown values. I have re-titled the columns to describe the physical quantities rather then their units. Quantities in bold are the answers. All answers are given to 4 sf.
| Voltage (V) \( V = I R \) |
Current (A) \( I = \frac{V}{R} \) |
Resistance (Ω) \( R = \frac{V}{I} \) |
|---|---|---|
| 25 | 10 | 2.5 |
| 45 | 5 | 9 |
| 1.5 | 1 | 1.5 |
| 72 | 6 | 12 |
| 1000 | 10 | 100 |
| 56 | 7 | 8 |
| 160 | 80 | 2 |
| 60 | 2 | 30 |
| 27 | 3 | 9 |
| 200 | 50 | 4 |
| 18 | 1.8 | 10 |
| 19 | 37 | 0.5135 |
Question 7
A. If the Resistance in a circuit is increased and the Voltage remains constant, Does the Current increase or decrease?
- The relevant Ohm's Law equation is \( I = \frac{V}{R} \). If \( R \) increases (assuming a constant voltage \( V \)), then \( I \) must decrease, since we are dividing by a larger number. The answer is decrease.
B. If the Voltage to a circuit increases and the Resistance remains constant, does the Current increase or decrease?
- The relevant Ohm's Law equation is \( I = \frac{V}{R} \). If \( V \) increases (assuming a constant resistance \( R \)), then \( I \) must increase, since we are multiplying by a larger number. The answer is increase.
C. If the Resistance to a circuit is doubled, then what has happened to the Voltage?
- The relevant Ohm's Law equation is \( V = I R \). If \( R \) increases (assuming a constant current \( I \)), then \( V \) must increase, since we are multiplying by a larger number. The answer is increase.