Electromagnetism Resource

Electromagnetic fields not only act in time, but also in space.

In particular, electromagnetic fields and forces not only have magnitude ("size", or "strength"), but also direction.

This makes electromagnetic fields vector fields.

Before introducing you to vectors, we must be familiar with scalar is.

Scalars

A scalar is a quantity that has a magnitude or value, but the direction is either meaningless or not important. Scalars include "ordinary" numbers, such as 1 or \( \pi \).

Some examples of scalars:

• time;
• length;
• area;
• volume;
• temperature;
• distance;
• speed;
• acceleration;
• electric charge;
• voltage;
• electric current;
• frequency.

In all these cases, the direction is not important.

Vectors and Vector Fields

See also: Vector (Simple English Wikipedia) :https://simple.wikipedia.org/wiki/Vector.

Vectors differ from scalars in that they have direction. The direction is what makes vectors useful for expressing effects that act in 3D space.

Many electromagnetic phenomena act in 3D space, so vectors are a useful way of expressing electromagnetic phenomena.

Examples of vectors:

• displacement (vector form of length and distance);
  
• velocity (vector form of speed);
  
• acceleration (when used as a vector);
• electric field;
• magnetic field;
• force (when used as a vector).
  

Vectors often occur as a vector field. Vector fields describe the nature of a vector (e.g. gravitational force) in space.

The image below shows an example of Earth's gravitational field, which is a vector field.

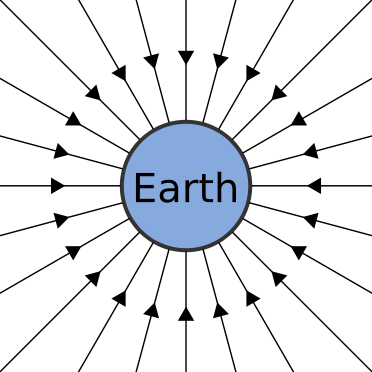
The arrows indicate the direction of Earth's gravitational force vector.

In this case, gravity has the effect of exerting a force that tries to pull an object with mass object towards the centre of the Earth.