Book C: SOHCAHTOA Worksheet 2 - Solutions and Commentary
Introduction
While the side lengths of right-angled triangles can be worked out using Pythagoras' Theorem, trigonometry and SOHCAHTOA provides a means of relating side lengths to angles in the triangle.
There are three main trigonometric functions: sine (\(\sin\)), cosine (\(\cos\)), and tangent (\(\tan\)).
The \(\sin\), \(\cos\) and \(tan\) functions are sometimes referred to as ratios, because they don't depend on the absolute lengths of the triangle, but the ratios of lengths. This means that it is only necessary to have one set of trigonometric
functions.
The figure below shows the relationship between side lengths and the angle \( \theta \) on a triangle embedded in a circle of radius 1. In this case, the hypotenuse has length 1, the side with length \( \cos \theta \) is adjacent to angle
\( \theta \), and the side with length \( \sin \theta \) is opposite to angle \( \theta \).
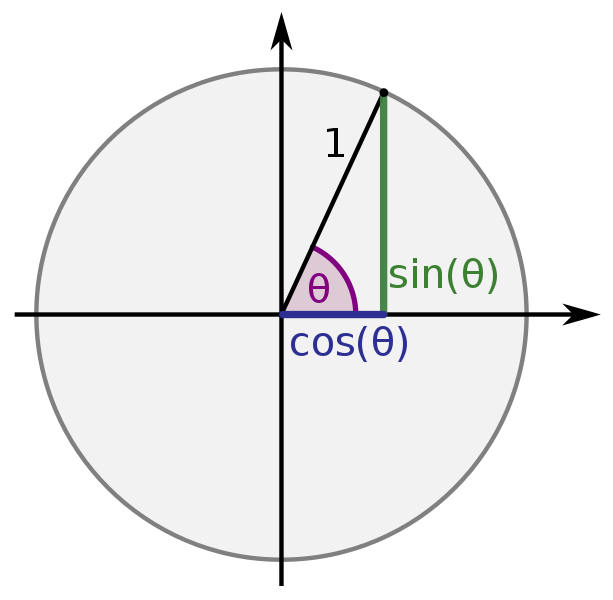
The tangent function relation is not shown, but it is the ratio of the lengths of the side \(\sin \theta\) to the length of the side \(\cos\theta\), i.e. \( \tan\theta = \frac{\sin\theta}{\cos\theta}\).
The circle diagram is important because every right angled triangle can be scaled to a right angled triangle with a hypotenuse length of 1. Or in other words, every right angled triangle is similar to a right-angled triangle with a hypotenuse
length of 1.
The figure below shows a right-angled triangle, with sides as marked. The opposite and adjacent sides are marked relative to angle A.
NOTE: The opposite and adjacent sides are swapped from the point of view of angle B.
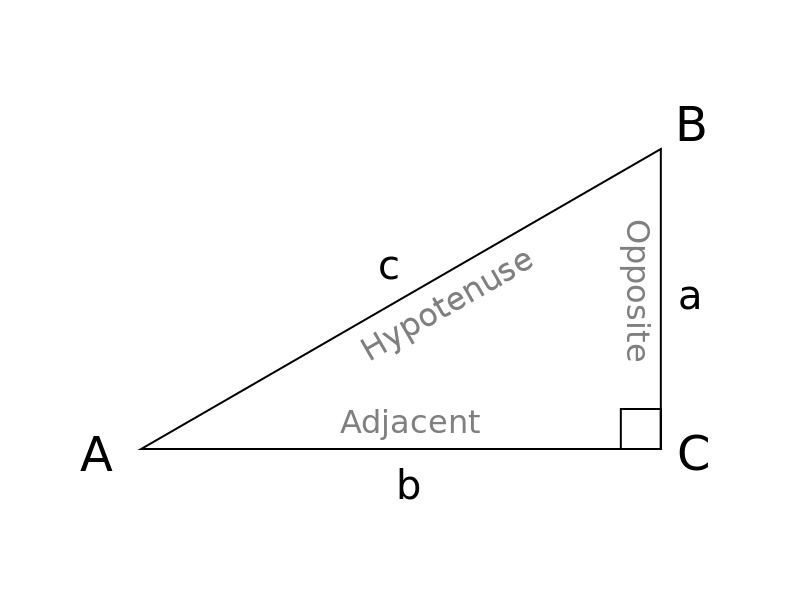
Note that angle A corresponds to angle \( \theta \) in the circle diagram above.
The SOHCAHTOA trigonometric relations are as follows, from the point of view of angle A.
SOH: \( \cos A = \frac{\mathrm{adj}}{\mathrm{hyp}} = \frac{b}{c} \)
CAH: \( \sin A = \frac{\mathrm{opp}}{\mathrm{hyp}} = \frac{a}{c} \)
TOA: \( \tan A = \frac{\mathrm{opp}}{\mathrm{adj}} = \frac{a}{b} \)
The SOHCAHTOA relations from the point of view of angle B are as follows. Notice that the \(\mathrm{adj}\), \(\mathrm{opp}\) and \(\mathrm{hyp}\) relations are the same, but the sides they correspond to are different.
SOH: \( \cos B = \frac{\mathrm{adj}}{\mathrm{hyp}} = \frac{a}{c} \)
CAH: \( \sin B = \frac{\mathrm{opp}}{\mathrm{hyp}} = \frac{b}{c} \)
TOA: \( \tan B = \frac{\mathrm{opp}}{\mathrm{adj}} = \frac{b}{a} \)
NOTE:
- When doing these calculations, make sure the angle unit on your calculator is set correctly. All these calculations assume your angle unit is degrees. A quick test is to perform the calculation \(\cos 90\). If the answer is zero, the angle is set correctly.
- It is always possible to check your answers by drawing the triangle to scale using a ruler and protractor, and measuring the sides.
- The hypotenuse is always the longest side, and opposite the 90° angle.
Solving for Angles
While the information above is useful for working out side lengths from a known angle, it does not provide any means for working out an unknown angle.
In other to calculate angles, we need to know about inverse trigonometric functions.
An inverse trigonometric function takes a ratio, and outputs an angle.
There are three main trigonometric functions: inverse sine (\(\sin^{-1}\)), inverse cosine (\(\cos^{-1}\)), and inverse tangent (\(\tan^{-1}\)). These may also be referred to as arc trigonometric functions e.g. arc sin, arc cos and arc tan.
The SOHCAHTOA trigonometric relations for the inverse functions are as follows, from the point of view of angle A.
SOH: \( A = \sin^{-1}\left(\frac{\mathrm{adj}}{\mathrm{hyp}}\right) = \sin^{-1}\left(\frac{b}{c}\right) \)
CAH: \( A = \cos^{-1}\left(\frac{\mathrm{opp}}{\mathrm{hyp}}\right) = \cos^{-1}\left(\frac{a}{c}\right) \)
TOA: \( A = \tan^{-1}\left(\frac{\mathrm{opp}}{\mathrm{adj}}\right) = \tan^{-1}\left(\frac{a}{b}\right) \)
The SOHCAHTOA relations from the point of view of angle B are as follows. Notice that the \(\mathrm{adj}\), \(\mathrm{opp}\) and \(\mathrm{hyp}\) relations are the same, but the sides they correspond to are different.
SOH: \( B = \sin^{-1}\left(\frac{\mathrm{adj}}{\mathrm{hyp}}\right) = \sin^{-1}\left(\frac{a}{c}\right) \)
CAH: \( B = \cos^{-1}\left(\frac{\mathrm{opp}}{\mathrm{hyp}}\right) = \cos^{-1}\left(\frac{b}{c}\right) \)
TOA: \( B = \tan^{-1}\left(\frac{\mathrm{opp}}{\mathrm{adj}}\right) = \tan^{-1}\left(\frac{b}{a}\right) \)
NOTE:
- When doing these calculations, make sure the angle unit on your calculator is set correctly. All these calculations assume your angle unit is degrees. A quick test is to perform the calculation \(\cos 90\). If the answer is zero, the angle is set correctly.
- It is always possible to check your answers by drawing the triangle to scale using a ruler and protractor, and measuring the angle with a protractor.
- The argument to \(\sin^{-1}\) and \(\cos^{-1}\) must always be in the range -1 to 1.
- Make sure the argument to \(\tan^{-1}\) is the correct ratio calculation. Otherwise the angle returned will be incorrect.
Question 1
The triangle is shown below, with the relevant sides labelled in terms of their relationship to the unknown angle \(\theta \).

The hypotenuse is 55 units long, and the side opposite the unknown angle is 22 units long, therefore the \(\sin^{-1}\) function is the relation we need.
\(\theta = \sin^{-1}\left(\frac{22}{55}\right) = \sin^{-1}\left(0.4\right) \) = 23.58° (2 dp) #.
Question 2
The triangle is shown below, with the relevant sides labelled in terms of their relationship to the unknown angle \(\theta \).
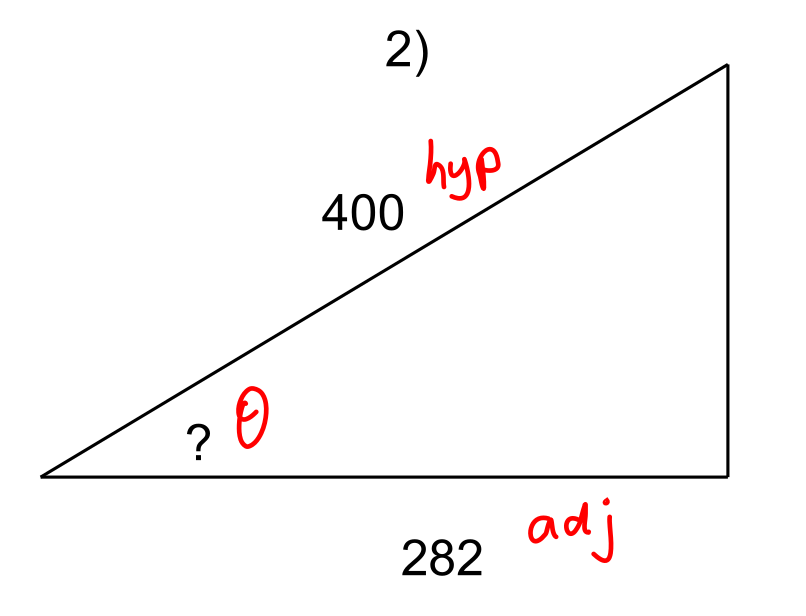
The hypotenuse is 400 units long, and the side adjacent the unknown angle is 282 units long, therefore the \(\cos^{-1}\) function is the relation we need.
\(\theta = \cos^{-1}\left(\frac{282}{400}\right) = \cos^{-1}\left(0.705\right) \) = 45.17° (2 dp) #.
Question 3
The triangle is shown below, with the relevant sides labelled in terms of their relationship to the unknown angle \(\theta \).
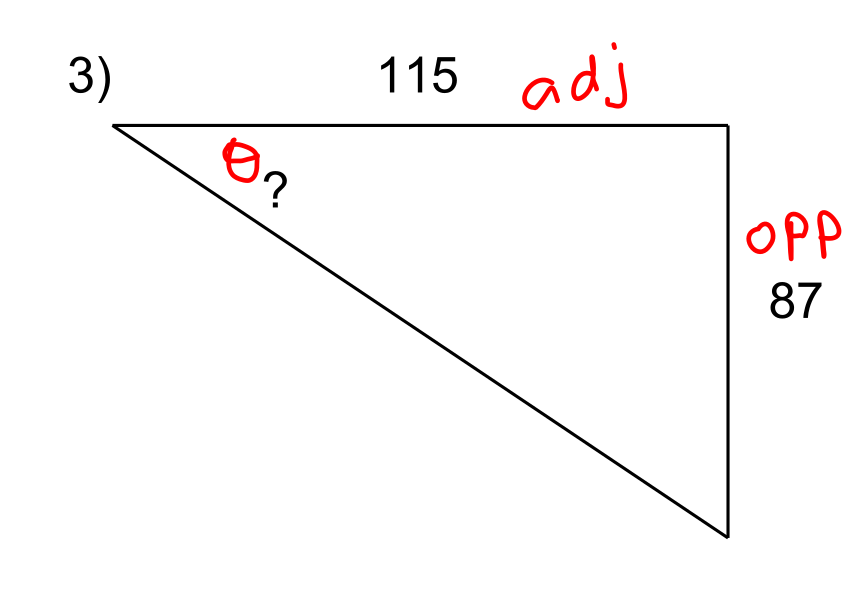
The side opposite the unknown angle is 87 units long, and the side adjacent the unknown angle is 115 units long, therefore the \(\tan^{-1}\) function is the relation we need.
\(\theta = \tan^{-1}\left(\frac{87}{115}\right) = \tan^{-1}\left(0.75652\right) \) = 37.11° (2 dp) #.
Question 4
The triangle is shown below, with the relevant sides labelled in terms of their relationship to the unknown angle \(\theta \).
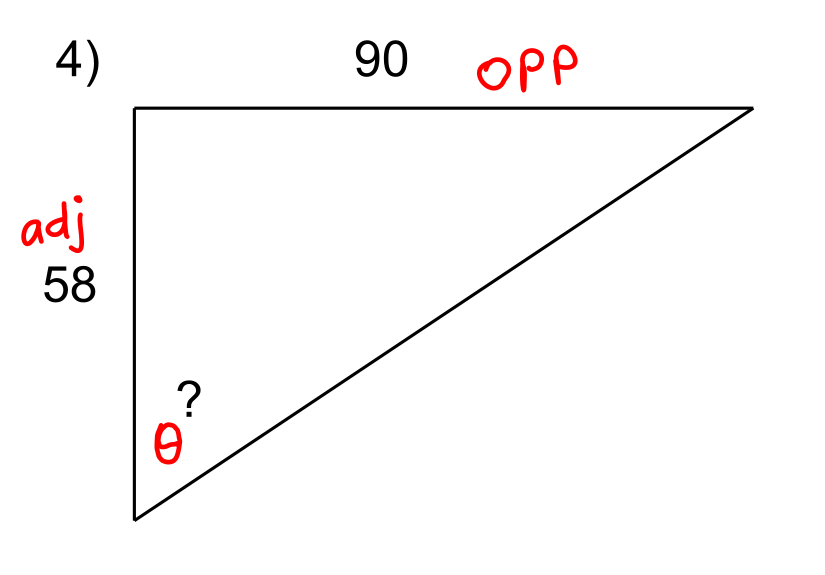
The side opposite the unknown angle is 90 units long, and the side adjacent the unknown angle is 58 units long, therefore the \(\tan^{-1}\) function is the relation we need.
\(\theta = \tan^{-1}\left(\frac{90}{58}\right) = \tan^{-1}\left(1.55172\right) \) = 57.20° (2 dp) #.
Question 5
The triangle is shown below, with the relevant sides labelled in terms of their relationship to the unknown angle \(\theta \).
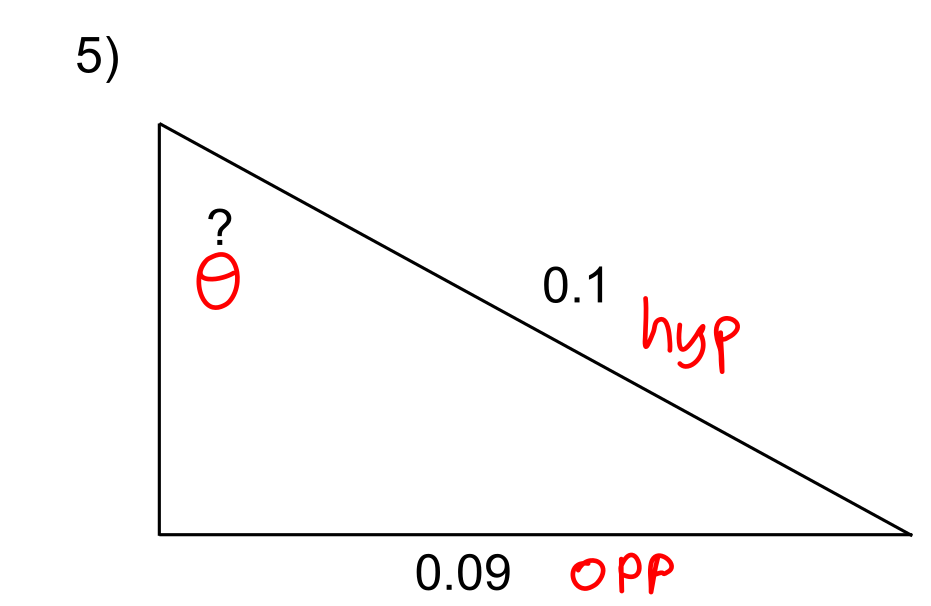
The hypotenuse is 0.1 units long, and the side opposite the unknown angle is 0.09 units long, therefore the \(\sin^{-1}\) function is the relation we need.
\(\theta = \sin^{-1}\left(\frac{0.09}{0.1}\right) = \sin^{-1}\left(0.9\right) \) = 64.16° (2 dp) #.